04 Navigation
Graph Intro#
- maze
-
tiles
-
Line Drawing
- Bresenham's Algorithm
- Linear Interpolation
Obstacles#
- Pure graph: 没有跨越障碍的边
- Tiles: keep a list to track
Single source, single destination#
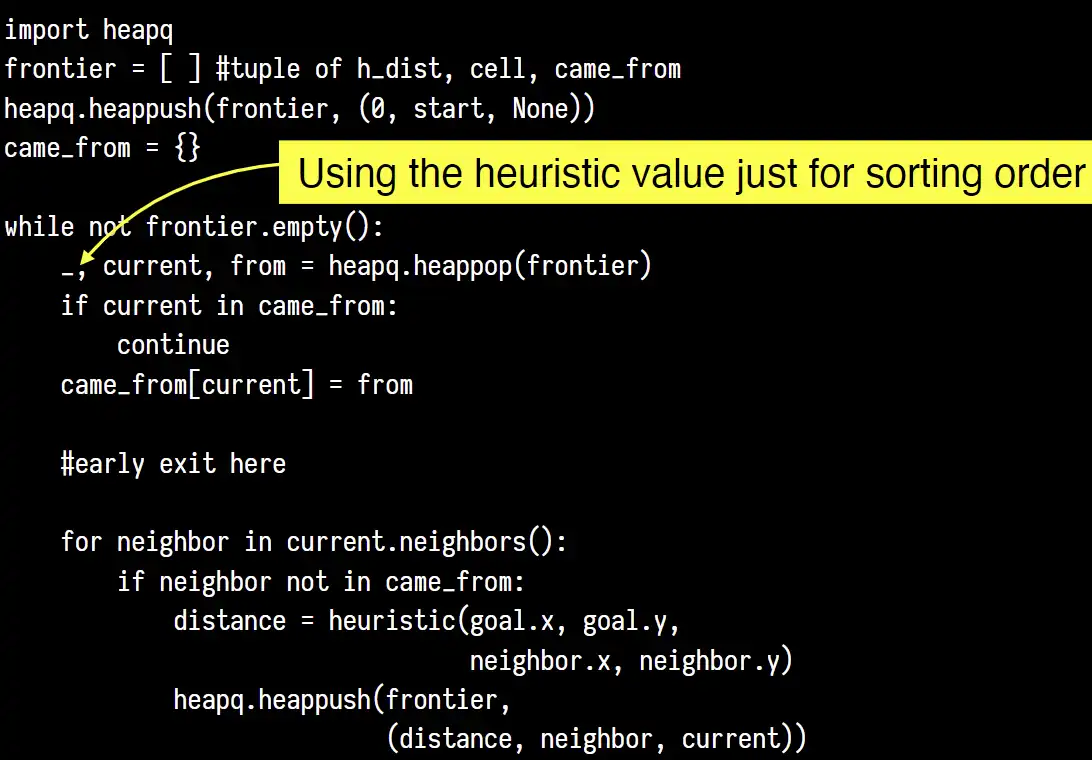
Greedy Best First Search#
比 single source, all destinations 会更快
Heuristic#
to guide our search
| L1 heuristic | |
|---|---|
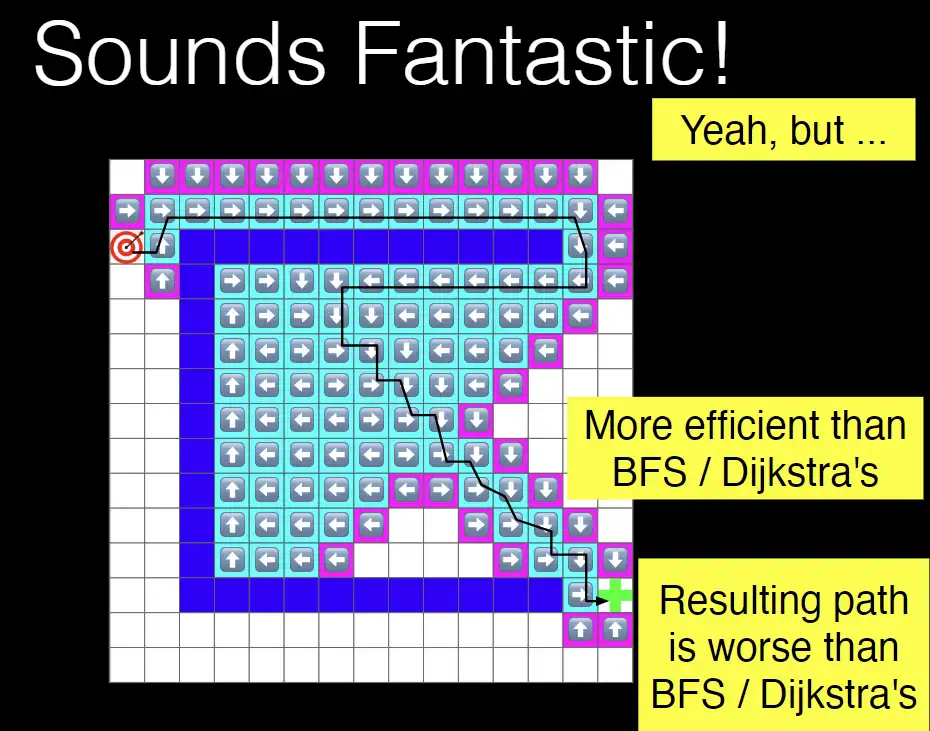
Warning
比 BFS/Dijkstra 更快,但是结果可能更差
pic
A*#
Games/Navigation/A-Star#
combining Dijkstra's and GBFS
{python}priority = cost + heuristic(goal.x, goal.y, neighbor.x, neighbor.y)- 将当前 cell 的 cost 和当前 cell 到目标的 heuristic cost 结合起来
- comments
- 如果 GBFS 有解,那么 A* 会探索完全相同的区域
- GBFS 可能会找到更长的路径,但是 A* 不会
- Dijkstra's 就是 A* 和常数 heuristic
- heuristic 能够帮助 A* 更快找到最优路径
Single source, all destinations#
BFS#
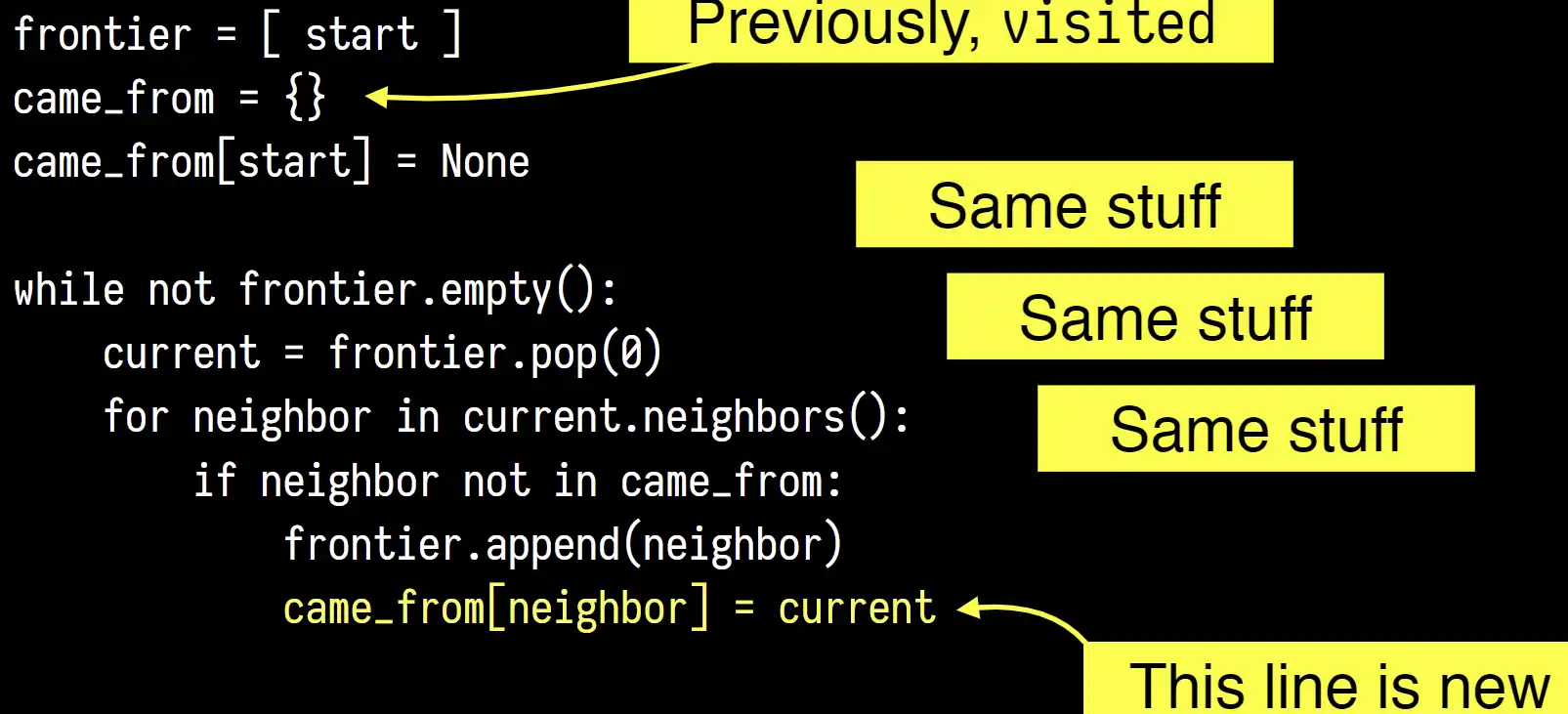
Algorithm/BFS#
unweighted edges
- Queue (FIFO)
- 保存反向指针来构建路径
early exit#
找到了目标就提前终止
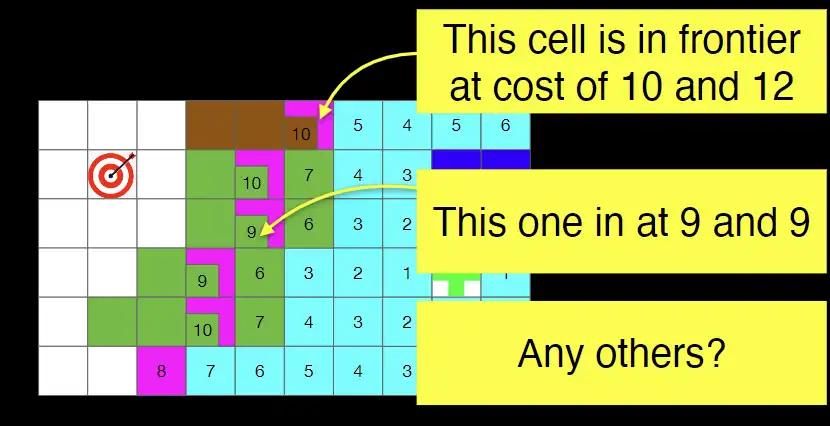
Dijkstra's#
- priority queue
some pics

Bellman-Ford#
cost can be negative
All sources, all destinations#
Floyd-Warshall Algorithm#
Johnson's Algirithm#
a mixture of Dijkstra's and Bellman-Ford
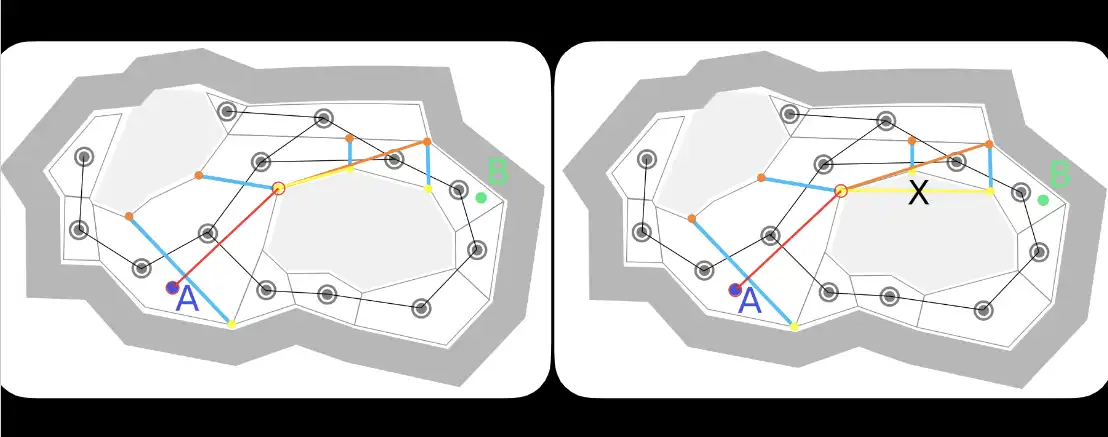
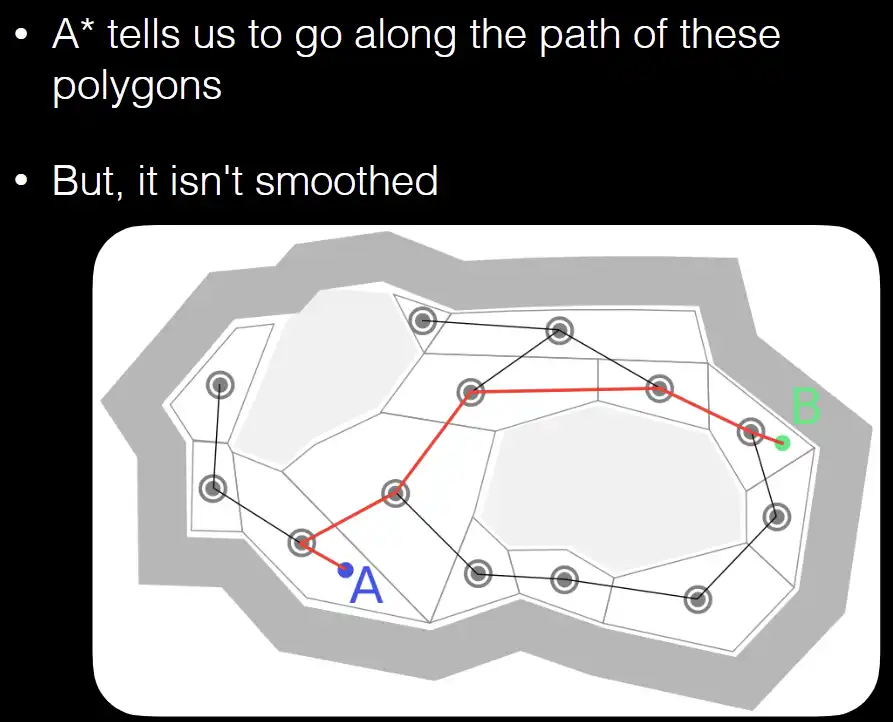
Navigation Meshes#
- Convex Polygons 凸多边形
- 在内部可以直接使用直线路径,走完之后仍然在其内部
- Navmesh
- 将可以使用的区域划分为凸多边形
- 每个多边形一定是 2d 的,但是整个地图不一定
algo#
- 如果 goal 和 agent 在同一个 polygon 内部,直线抵达
- 否则将 polygon 映射成 graph,使用 A*,最后再用 rule #1
Funnel Algorithm#
navmesh 过于简化了真实情况,如果有很大的 polygon,可能需要花很多时间到这个 polygon 的中心
- 首先根据 A* 算法得到将要经过的 polygons,接下来关注 polygons 的邻接边
- 考虑跟踪邻接边的左侧和右侧
- 当下一步可以从上一个拐点直线连接时,直线连接
- 如果不能,找最近的新拐点,再直线连接
- 最后一步就是到终点,然后选择 cost 较小的一条